Defining One Solution in Algebra: A Mathematical Explanation

_{October 1, 2023 by JoyAnswer.org, Category : Mathematics}

What is the definition of one solution in Algebra? Gain a clear understanding of the mathematical concept of "one solution" in algebraic equations and its significance in problem-solving.

Table of Contents

What is the definition of one solution in Algebra?

In algebra, when we talk about "one solution," we are referring to the outcome of solving an equation or a system of equations, and it means that there is a single unique value for the variable(s) that satisfies the equation(s).

Here are a few key points to understand about one solution in algebra:

Equations: An equation is a mathematical statement that asserts that two expressions are equal. For example, the equation "2x + 3 = 7" states that the expression "2x + 3" is equal to the expression "7."
Solution: A solution to an equation is a value or set of values for the variable(s) that make the equation true. In the equation "2x + 3 = 7," the value of x that makes this equation true is x = 2, because when you substitute 2 for x, you get: 2(2) + 3 = 7, which is true.
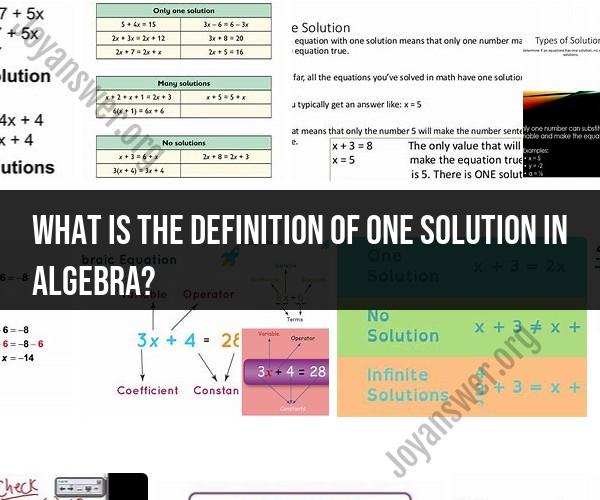
One Solution: An equation has one solution when there is only a single value for the variable that satisfies the equation. In other words, there is only one value that makes the equation true. This is often represented as a specific number or as a specific set of values for multiple variables.

For example:

The equation "3x - 5 = 4" has one solution, which is x = 3. When you substitute 3 for x, you get: 3(3) - 5 = 9 - 5 = 4, which is true.
The system of equations:
- 2x + y = 5
- x - 3y = 2has one solution, which is x = 2 and y = 1. When you substitute these values into both equations, they are satisfied.

It's important to note that not all algebraic equations or systems of equations have one solution. Some may have no solutions (inconsistent) or infinitely many solutions (dependent or coincident equations). The uniqueness of the solution depends on the specific equations and variables involved.

1. Algebra Unveiled: Defining "One Solution" in Mathematical Terms

In mathematics, a one-solution equation is an equation that has exactly one solution for the unknown variable. This means that there is only one value for the variable that makes the equation true.

One-solution equations can be written in a variety of forms, but they all have one thing in common: they are all solvable. This means that there is a mathematical process that can be used to find the solution to the equation.

Some examples of one-solution equations include:

Linear equations, such as 2x + 3 = 7
Quadratic equations, such as x^2 - 3x + 2 = 0
Rational equations, such as \frac{3x}{x + 1} = 2

Solving for Certainty: The Algebraic Concept of a Single Solution

The algebraic concept of a single solution is important because it allows us to solve equations with confidence. When we know that an equation has exactly one solution, we can be sure that the answer we get is correct.

There are a number of different ways to solve equations, but they all rely on the same basic principle: to isolate the unknown variable on one side of the equation. Once the unknown variable is isolated, we can solve for its value.

For example, to solve the equation 2x + 3 = 7, we would first subtract 3 from both sides of the equation. This gives us:

2x = 4

Next, we would divide both sides of the equation by 2. This gives us our solution:

x = 2

We can check our answer by substituting x = 2 back into the original equation:

2(2) + 3 = 7

4 + 3 = 7

7 = 7

Since both sides of the equation are equal, we know that our answer is correct.

3. When Algebra Speaks of Unity: The Definition of "One Solution"

The definition of "one solution" in algebra is a fundamental concept that is essential for understanding and solving equations. When algebra speaks of unity, it is referring to the idea that there is only one value for the unknown variable that makes the equation true.

This concept is important because it allows us to solve equations with confidence. When we know that an equation has exactly one solution, we can be sure that the answer we get is correct.

The definition of "one solution" is also important because it allows us to develop and use mathematical models. Mathematical models are representations of real-world phenomena that are used to make predictions and solve problems. In order to be accurate, mathematical models must be based on equations that have one solution.

For example, consider the following mathematical model of a falling object:

s = 1/2 * g * t^2

where:

s is the distance fallen in meters
g is the acceleration due to gravity (9.81 m/s^2)
t is the time in seconds

This model has exactly one solution for the distance fallen, given the time. This means that we can use the model to predict the distance that an object will fall after a given amount of time, or to solve for the time it will take for an object to fall a given distance.

The definition of "one solution" is a powerful concept that is essential for understanding and using algebra. It allows us to solve equations with confidence and to develop and use mathematical models to solve real-world problems.