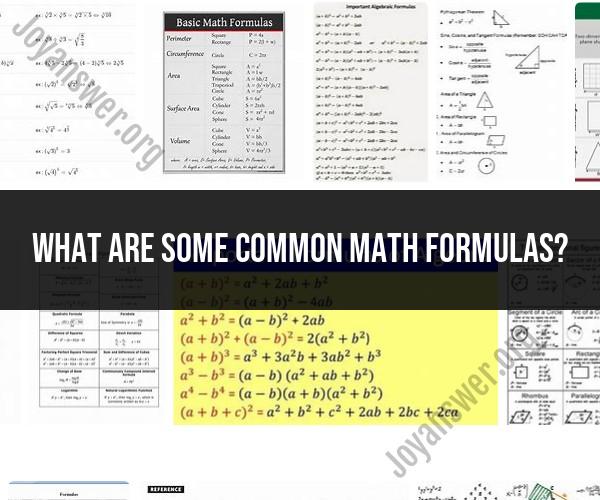
Common Math Formulas: Everyday Mathematical Tools

_{October 17, 2023 by JoyAnswer.org, Category : Mathematics}

What are some common math formulas? Explore a compilation of common math formulas that are frequently used in everyday mathematical calculations and problem-solving.

$Common Math Formulas: Everyday Mathematical Tools$

What are some common math formulas?

Mathematics plays a crucial role in our daily lives, and many common math formulas are used regularly for various tasks. Here are some everyday mathematical tools and formulas:

Basic Arithmetic:
- Addition: a + b = c
- Subtraction: a - b = c
- Multiplication: a * b = c
- Division: a / b = c
Percentages:
- Percentage Change: ((New Value - Old Value) / Old Value) * 100%
- Percentage of a Number: (Percentage / 100) * Number
Geometry:
- Area of a Rectangle: Length * Width
- Area of a Triangle: (1/2) * Base * Height
- Area of a Circle: π * (Radius^2)
- Circumference of a Circle: 2 * π * Radius
- Volume of a Cylinder: π * (Radius^2) * Height
Algebra:
- Quadratic Formula: (-b ± √(b^2 - 4ac)) / (2a)
- Slope-Intercept Form (for a linear equation): y = mx + b (m is the slope, b is the y-intercept)
Interest and Finance:
- Simple Interest: I = P * R * T (Interest = Principal × Rate × Time)
- Compound Interest: A = P(1 + (r/n))^(nt) (A is the final amount, P is the principal, r is the annual interest rate, n is the number of times interest is compounded per year, and t is the number of years)
Statistics:
- Mean (Average): (Sum of Data Points) / (Number of Data Points)
- Median: Middle value in a dataset (or average of the two middle values for an even number of data points)
- Standard Deviation: A measure of data dispersion
- Variance: The square of the standard deviation
Probability:
- Probability of an Event: P(A) = (Number of Favorable Outcomes) / (Total Number of Outcomes)
- Probability of Independent Events: P(A and B) = P(A) * P(B)
Trigonometry:
- Sine (sin): Opposite / Hypotenuse
- Cosine (cos): Adjacent / Hypotenuse
- Tangent (tan): Opposite / Adjacent
- Pythagorean Theorem: a^2 + b^2 = c^2 (relates the sides of a right triangle)
Logarithms:
- Logarithm of a Number: log_b(a) = c (b is the base, a is the number, and c is the exponent to which the base must be raised to get the number a)

These are just a few examples of common math formulas and tools that people use in everyday life. They are essential for solving a wide range of problems, from budgeting and cooking to home improvement and problem-solving in various professions.

1. Common mathematical formulas for everyday use

Some common mathematical formulas for everyday use include:

Area of a rectangle: length * width
Perimeter of a rectangle: 2 * (length + width)
Area of a triangle: 1/2 * base * height
Perimeter of a triangle: side 1 + side 2 + side 3
Volume of a rectangular prism: length * width * height
Distance formula: sqrt((x2 - x1)^2 + (y2 - y1)^2)
Simple interest formula: principal * interest rate * time
Compound interest formula: principal * (1 + interest rate)^time

These formulas can be used to solve a variety of everyday problems, such as calculating the area of a room, the distance between two points, or the amount of interest earned on a savings account.

2. How mathematical formulas simplify problem-solving in various fields

Mathematical formulas simplify problem-solving in various fields by providing a concise and efficient way to represent complex relationships. For example, the distance formula can be used to calculate the distance between two points on a map, the Pythagorean theorem can be used to solve right triangles, and the quadratic formula can be used to solve quadratic equations.

Mathematical formulas are also essential for many scientific and engineering applications. For example, the laws of physics are expressed in mathematical terms, and engineers use mathematical formulas to design and build everything from bridges to airplanes to computer chips.

3. Fundamental math formulas for geometry and algebra

Some fundamental math formulas for geometry and algebra include:

Geometry:
- Pythagorean theorem: a^2 + b^2 = c^2
- Distance formula: sqrt((x2 - x1)^2 + (y2 - y1)^2)
- Area of a triangle: 1/2 * base * height
- Area of a rectangle: length * width
- Volume of a rectangular prism: length * width * height
Algebra:
- Quadratic formula: x = (-b +/- sqrt(b^2 - 4ac)) / 2a
- Slope-intercept form of a linear equation: y = mx + b
- Distance formula: sqrt((x2 - x1)^2 + (y2 - y1)^2)
- Pythagorean theorem: a^2 + b^2 = c^2
- Law of cosines: c^2 = a^2 + b^2 - 2ab * cos(C)
- Law of sines: a / sin(A) = b / sin(B) = c / sin(C)

These formulas can be used to solve a variety of geometry and algebra problems, such as calculating the area or volume of a shape, finding the distance between two points, or solving for the roots of a quadratic equation.

4. Applications of mathematical formulas in science and engineering

Mathematical formulas are essential for many scientific and engineering applications. Here are a few examples:

Physics: Newton's laws of motion, the laws of thermodynamics, and the laws of electromagnetism are all expressed in mathematical terms. These formulas are used to design and build everything from bridges to airplanes to computer chips.
Chemistry: Mathematical formulas are used to calculate the properties of molecules and compounds, and to design and conduct chemical experiments.
Biology: Mathematical formulas are used to model and analyze biological systems, such as the spread of diseases and the growth of populations.
Engineering: Engineers use mathematical formulas to design and build bridges, roads, buildings, and other infrastructure. They also use mathematical formulas to design and manufacture products, such as cars, airplanes, and computers.

5. Resources or tools for quickly accessing essential math formulas

There are a number of resources and tools available for quickly accessing essential math formulas. Some of these include:

Math websites and apps: There are a number of websites and apps that provide access to a wide range of mathematical formulas. Some of these resources include MathWorld, WolframAlpha, and Symbolab.
Math textbooks and reference books: Math textbooks and reference books are another good source for finding mathematical formulas.
Math teachers and tutors: Math teachers and tutors can also be a valuable resource for finding and understanding mathematical formulas.

If you are ever unsure of where to find a particular mathematical formula, you can always do a quick Google search. There are a number of websites that provide easy-to-understand explanations of mathematical formulas and examples of how to use them.