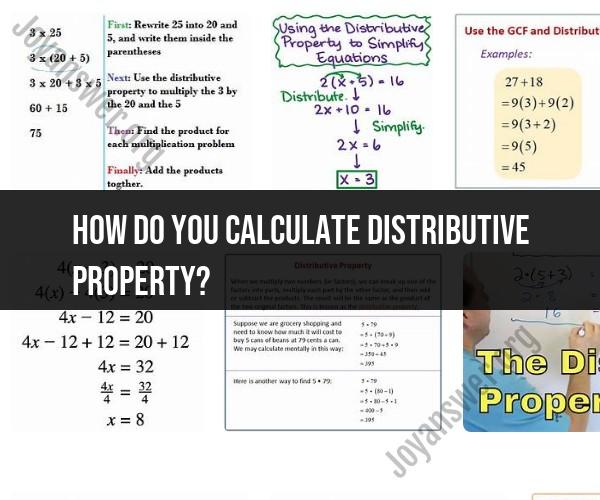
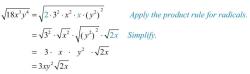Calculating with the Distributive Property: Step-by-Step Guide

_{October 17, 2023 by JoyAnswer.org, Category : Mathematics}

How do you calculate distributive property? Follow a step-by-step guide on how to calculate using the distributive property, an essential skill in algebra and mathematics.

Table of Contents

How do you calculate distributive property?

Calculating with the distributive property involves using the property to simplify or expand algebraic expressions or perform mathematical operations efficiently. Here is a step-by-step guide on how to calculate with the distributive property:

Step 1: Understand the Distributive Property:Before you start calculating, ensure you understand the distributive property. It states that for any numbers a, b, and c:

a * (b + c) = (a * b) + (a * c)

Step 2: Identify the Expression:Start with the expression that you want to simplify or expand using the distributive property. It may look like a * (b + c), (a + b) * (c + d), or similar expressions.

Step 3: Determine the Values:Know the values of a, b, and c (or other variables) in your expression. You need these values to perform the calculation.

Step 4: Apply the Distributive Property:Here's how to use the distributive property in different scenarios:

Simplifying Expressions:

If you want to simplify an expression like a * (b + c), distribute the 'a' to both 'b' and 'c.' For example, if a = 3, b = 2, and c = 4:a * (b + c) = 3 * (2 + 4) = 3 * 2 + 3 * 4 = 6 + 12 = 18

Expanding Expressions:

If you need to expand an expression like (a + b) * (c + d), apply the distributive property to both terms inside the parentheses. For example:(a + b) * (c + d) = a * c + a * d + b * c + b * d

Factoring Expressions:

When factoring an expression, you use the distributive property to factor out a common factor from all terms. For example, to factor 2 out of 2x + 4y:2(x + 2y)

Solving Equations:

If you're solving an equation, use the distributive property to isolate variables or simplify the equation. For example, in the equation 3(x + 2) = 21:3(x + 2) = 213x + 6 = 21

Step 5: Simplify the Result:Once you've applied the distributive property and performed the necessary calculations, simplify the expression or equation further if possible. This may involve combining like terms, solving for variables, or evaluating numerical expressions.

Step 6: Check Your Work:After simplifying or expanding an expression, it's essential to check your work for accuracy. Ensure that you've correctly applied the distributive property and performed all calculations accurately.

Step 7: Practice:The more you practice using the distributive property, the more proficient you'll become. Practice with a variety of examples to build your skills and confidence in using this important mathematical property.

By following these steps and practicing with different expressions, you can effectively use the distributive property to simplify or expand algebraic expressions, solve equations, and perform various mathematical operations.

How do you calculate using the distributive property in mathematics?

To calculate using the distributive property in mathematics, follow these steps:

Identify the expression or equation that you want to simplify.
Look for the number that is being multiplied by the sum or difference.
Distribute the number to each term of the sum or difference.
Combine the like terms.
Simplify the expression or equation.

Can you provide step-by-step examples of performing calculations with the distributive property?

Here are some step-by-step examples of performing calculations with the distributive property:

Example 1:

Simplify the expression $5 (2 + 3)$ using the distributive property.

Solution:

Identify the expression that we want to simplify: $5 (2 + 3)$
Look for the number that is being multiplied by the sum: $5$
Distribute the number to each term of the sum: $5 (2 + 3) = 5 (2) + 5 (3)$
Combine the like terms: $5 (2) + 5 (3) = 10 + 15$
Simplify the expression: $10 + 15 = 25$

Example 2:

Solve the multiplication problem $5 \times (20 - 10)$ using the distributive property.

Solution:

Identify the multiplication problem that we want to solve: $5 \times (20 - 10)$
Look for the number that is being multiplied by the sum: $5$
Distribute the number to each term of the sum: $5 \times (20 - 10) = 5 \times (20) - 5 \times (10)$
Combine the like terms: $5 \times (20) - 5 \times (10) = 100 - 50$
Simplify the expression: $100 - 50 = 50$

How to apply the distributive property to simplify algebraic expressions and equations?

To apply the distributive property to simplify algebraic expressions and equations, follow the same steps as for simplifying numerical expressions. For example, to simplify the algebraic expression $5 (2 x + 3 y)$ , we would follow these steps:

Identify the expression that we want to simplify: $5 (2 x + 3 y)$
Look for the number that is being multiplied by the sum: $5$
Distribute the number to each term of the sum: $5 (2 x + 3 y) = 5 (2 x) + 5 (3 y)$
Combine the like terms: $5 (2 x) + 5 (3 y) = 10 x + 15 y$
Simplify the expression: $10 x + 15 y = 10 x + 15 y$

We can also use the distributive property to simplify equations. For example, to simplify the equation $5 (2 x + 3) = 10$ , we would follow these steps:

Distribute the number in the left-hand side of the equation: $5 (2 x + 3) = 10$
Combine the like terms: $10 x + 15 = 10$
Subtract 15 from both sides of the equation: $10 x = - 5$
Divide both sides of the equation by 10: $x = - \frac{1}{2}$

What are common mistakes or challenges to avoid when using the distributive property?

Here are some common mistakes or challenges to avoid when using the distributive property:

Not distributing the number to each term of the sum or difference.
Not combining like terms.
Making careless mistakes when simplifying the expression or equation.

To avoid these mistakes, it is important to be careful and to double-check your work.

How to gain proficiency in using the distributive property effectively?

The best way to gain proficiency in using the distributive property effectively is to practice using it. Here are some tips:

Solve a variety of problems that involve the distributive property.
Pay attention to the patterns that you see when using the distributive property.
Look for opportunities to use the distributive property in real-world problems.

With practice, you will become proficient in using the distributive property to solve a variety of problems.