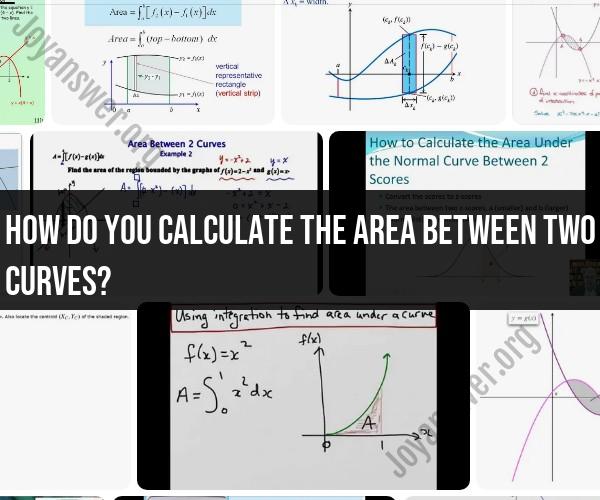
Calculating the Area Between Two Curves: Geometric Analysis

_{October 21, 2023 by JoyAnswer.org, Category : Mathematics}

How do you calculate the area between two curves? Explore the techniques and methods for calculating the area between two curves, a valuable skill in mathematical analysis.

Table of Contents

How do you calculate the area between two curves?

Calculating the area between two curves is a common problem in calculus. The key is to find the difference between the integrals of the two functions over a specified interval. Here are the steps to calculate the area between two curves:

Step 1: Identify the Intersection PointsBefore you can find the area between two curves, you need to determine where the two curves intersect. These are the x-values at which the two functions are equal. Set the two functions equal to each other and solve for x to find these points.

For example, if you have two functions, f(x) and g(x), you would solve the equation:f(x) = g(x)

The solutions to this equation represent the x-values where the two curves intersect.

Step 2: Determine the IntervalIdentify the interval over which you want to find the area between the two curves. This interval is typically determined by the x-values where the curves intersect. It could be from one intersection point to another or between more complex intersections, depending on the problem.

Step 3: Set Up the IntegralTo calculate the area between the two curves, you'll need to set up an integral that subtracts one function from the other over the specified interval. The formula is as follows: $Area = \int_{a}^{b} \left| f(x) - g(x) \right| dx$

Here, "a" and "b" are the x-values of the intersection points that define the interval. The absolute value (| |) ensures that the area is positive, even if one function is above the other in certain regions.

Step 4: Calculate the IntegralUse the fundamental theorem of calculus to evaluate the integral: $Area = \int_{a}^{b} \left| f(x) - g(x) \right| dx$

Integrate the absolute difference function from "a" to "b" to find the area between the two curves.

Step 5: Interpret the ResultThe value you obtain from the integral represents the area between the two curves. If the value is positive, it means the area is above the x-axis. If it's negative, it means the area is below the x-axis.

Keep in mind that the choice of which curve is subtracted from the other in the absolute difference affects the sign of the result. It's essential to set up the integral correctly based on your problem's context.

This method works for finding the area between two curves in a standard xy-plane. If the problem involves polar coordinates or other coordinate systems, the approach may differ. Additionally, the method can be adapted for finding the area between curves and the x-axis by using the absolute value of a single function (|f(x)|) in the integral.

Calculating the Area Between Two Curves: A Mathematical Guide

The area between two curves is a fundamental concept in calculus with a wide range of applications in science and engineering. It is defined as the region bounded by the two curves and the x-axis.

The Concept of Area Between Curves in Calculus

In calculus, the area between two curves can be calculated using the definite integral. The definite integral is a type of integral that calculates the area under a curve over a closed interval.

To calculate the area between two curves using the definite integral, we first need to define the region that we want to calculate the area of. This can be done by sketching the two curves on a graph and shading in the region that we want to calculate the area of.

Once we have defined the region, we can then calculate the area using the following formula:

∫ba(f(x)−g(x))dx

where:

f(x) and g(x) are the two curves
[a, b] is the interval over which we want to calculate the area

Integrating Functions and Defining the Bounded Region

To integrate the functions and define the bounded region, we need to first identify the two functions that define the curves and the interval over which we want to calculate the area.

Once we have identified the functions and the interval, we can then use the definite integral formula to calculate the area.

Solving Real-World Problems Using Area Between Curves

The area between two curves can be used to solve a variety of real-world problems. For example, it can be used to calculate the:

Work done by a force
Heat transferred by conduction
Volume of a solid of revolution
Flow rate of a fluid

Advanced Techniques and Applications of Area Calculation

There are a variety of advanced techniques and applications of area calculation. For example, the area between two curves can be used to:

Calculate the center of mass of a solid
Calculate the moment of inertia of a solid
Calculate the surface area of a solid
Calculate the volume of a solid enclosed by multiple surfaces

Conclusion

The area between two curves is a powerful tool that can be used to solve a variety of problems in mathematics, science, and engineering. By understanding the concept of area between curves and the definite integral formula, you can learn to calculate the area between two curves and apply it to solve real-world problems.