Calculating a Linear Equation: Step-by-Step Approach

_{November 7, 2023 by JoyAnswer.org, Category : Mathematics}

How do you calculate a linear equation? Get a step-by-step approach to calculate a linear equation and understand the mathematical concepts involved.

Table of Contents

How do you calculate a linear equation?

Calculating or determining a linear equation involves finding the equation that represents a straight line on a graph. A linear equation is typically written in the form:

$y = mx + b$

Where:

$y$ is the dependent variable (usually representing the vertical axis on a graph).
$x$ is the independent variable (usually representing the horizontal axis on a graph).
$m$ is the slope of the line, which represents the rate of change or how steep the line is.
$b$ is the y-intercept, which is the value of $y$ when $x$ is equal to 0. It represents where the line intersects the y-axis.

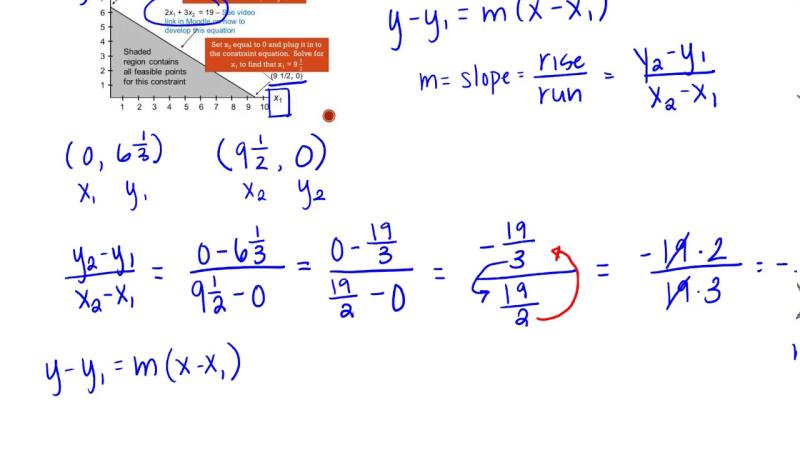
To calculate a linear equation, you typically need two pieces of information: a point on the line (x, y) and the slope ( $m$ ) of the line. Here's a step-by-step approach:

Find the Slope ( $m$ ):
- If you have two points on the line, you can use the following formula to calculate the slope: $m = \frac{y_2 - y_1}{x_2 - x_1}$
- If you have the slope ( $m$ ) given, you can skip this step.
Write the Equation in Slope-Intercept Form:
- Use the slope ( $m$ ) and a point ( $x_1, y_1$ ) on the line to write the equation in slope-intercept form: $y - y_1 = m(x - x_1)$
- Then, solve for $y$ to get the equation in the form $y = mx + b$ .
Determine the Y-Intercept ( $b$ ):
- Substitute the coordinates of the point ( $x_1, y_1$ ) into the equation from step 2 and solve for $b$ : $y_1 = m(x_1) + b$
- Solve for $b$ , which is the y-intercept.
Write the Linear Equation:
- Use the values of $m$ and $b$ to write the equation in the form $y = mx + b$ .

Here's an example:

Suppose you have a line with a slope of 2 and it passes through the point (3, 5).

Find the Slope: $m = 2$
Write the Equation in Slope-Intercept Form: $y - 5 = 2(x - 3)$
Determine the Y-Intercept: $5 = 2(3) + b$ $5 = 6 + b$ $b = -1$
Write the Linear Equation: $y = 2x - 1$

This is the equation of the line with a slope of 2 and a y-intercept of -1.

Calculating Linear Equations: Basics and Techniques

Linear equations are fundamental mathematical expressions that represent a balance between two quantities. They are commonly represented in the form of Ax + B = C, where A, B, and C are constants and x is the variable to be solved for.

Basic Techniques for Solving Linear Equations:

Addition and Subtraction: Add or subtract the same number to both sides of the equation to isolate the variable.
Multiplication and Division: Multiply or divide both sides of the equation by the same non-zero number to isolate the variable.
Combining Like Terms: Combine like terms on the same side of the equation to simplify the expression.
Transposing Terms: Move terms from one side of the equation to the other by adding or subtracting them from both sides.
Substitution: Substitute known values for variables to solve for other unknown variables.

How to Solve Linear Equations Successfully

To solve linear equations successfully, follow these steps:

Read and Understand the Problem: Carefully read the problem to identify the given information and what you need to solve for.
Write the Equation: Translate the problem into a mathematical equation of the form Ax + B = C.
Isolate the Variable: Apply the appropriate technique to isolate the variable x on one side of the equation.
Solve for the Variable: Simplify the expression and perform any necessary calculations to find the value of x.
Verify the Solution: Check if the obtained solution satisfies the original equation and makes sense in the context of the problem.

Understanding the Principles of Linear Equation Solution

The principles behind solving linear equations involve manipulating the equation to isolate the variable and determine its value. These manipulations are based on the following properties:

Equality Property: If two expressions are equal, you can add or subtract the same quantity to both sides without affecting the equality.
Multiplication Property: If an expression is equal to another expression, you can multiply or divide both sides by the same non-zero quantity without affecting the equality.
Combining Like Terms Property: Like terms can be combined on the same side of the equation by adding or subtracting their coefficients.
Transposition Property: Any quantity can be moved from one side of the equation to the other by adding or subtracting it from both sides, effectively changing its sign.
Substitution Property: If an expression has a known value, you can substitute that value wherever the expression appears in the equation.