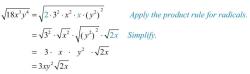Matrix Operations: Understanding Mathematical Manipulations

What is matrix operations?

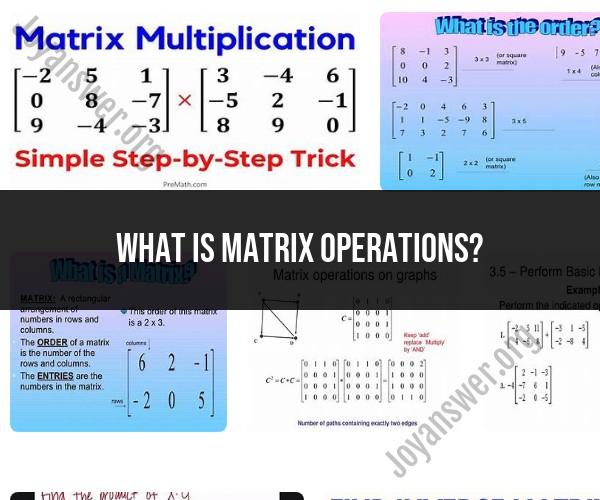
Matrix operations refer to various mathematical manipulations or calculations that can be performed on matrices, which are rectangular arrays of numbers, symbols, or expressions arranged in rows and columns. These operations allow mathematicians, scientists, engineers, and computer scientists to perform a wide range of tasks, including solving systems of linear equations, transforming data, and conducting various mathematical analyses.

The fundamental matrix operations include:

1. Matrix Addition: In matrix addition, corresponding elements in two matrices are added together. This operation is only defined for matrices of the same dimensions (i.e., they must have the same number of rows and columns). The result is another matrix with the same dimensions.

   Example:

   $$\begin{bmatrix}1 & 2 \\3 & 4\end{bmatrix}+\begin{bmatrix}5 & 6 \\7 & 8\end{bmatrix}=\begin{bmatrix}6 & 8 \\10 & 12\end{bmatrix}$$

2. Matrix Subtraction: Similar to matrix addition, matrix subtraction involves subtracting corresponding elements in two matrices. As with addition, the matrices must have the same dimensions, and the result is another matrix of the same dimensions.

   Example:

   $$\begin{bmatrix}5 & 6 \\7 & 8\end{bmatrix}-\begin{bmatrix}1 & 2 \\3 & 4\end{bmatrix}=\begin{bmatrix}4 & 4 \\4 & 4\end{bmatrix}$$

3. Scalar Multiplication: Scalar multiplication involves multiplying a matrix by a single number (scalar). Each element in the matrix is multiplied by the scalar value.

   Example:

   $$2 \times\begin{bmatrix}1 & 2 \\3 & 4\end{bmatrix}=\begin{bmatrix}2 & 4 \\6 & 8\end{bmatrix}$$

4. Matrix Multiplication: Matrix multiplication is a more complex operation. It involves multiplying two matrices together following specific rules. In matrix multiplication, the number of columns in the first matrix must be equal to the number of rows in the second matrix. The result is a new matrix with dimensions determined by the original matrices.

   Example:

   $$\begin{bmatrix}1 & 2 \\3 & 4\end{bmatrix}\times\begin{bmatrix}5 & 6 \\7 & 8\end{bmatrix}=\begin{bmatrix}19 & 22 \\43 & 50\end{bmatrix}$$

Matrix operations are fundamental in various fields of mathematics, physics, engineering, computer science, and data analysis. They are used for solving linear equations, representing transformations in computer graphics, analyzing data in statistics, and much more. Understanding and mastering matrix operations are essential for professionals working in these fields.