Mastering Integral Rules: Techniques for Calculus Success

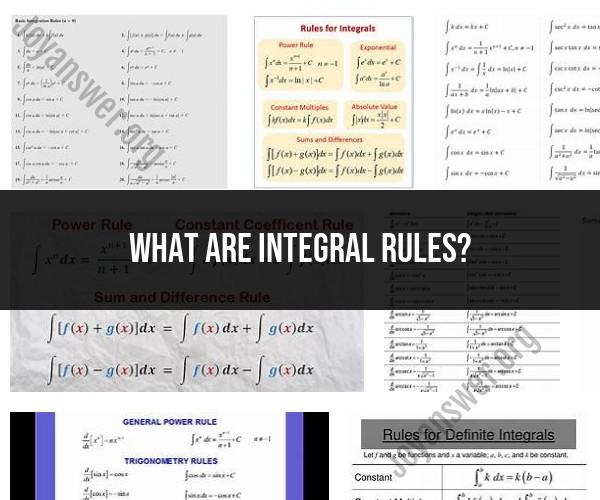
Integral rules are a set of fundamental principles and techniques used in calculus to evaluate and solve definite and indefinite integrals. Integrals are mathematical operations that involve finding the antiderivative of a function, which represents the accumulation of a quantity over a specified interval. Understanding integral rules is crucial for success in calculus. Here are some important integral rules and techniques:

1. Power Rule: The power rule is used for integrating monomials. For a function of the form ∫x^n dx, where n is not equal to -1, the integral is given by:∫x^n dx = (x^(n+1))/(n+1) + C, where C is the constant of integration.

2. Constant Rule: The integral of a constant function is simply the constant times the variable:∫k dx = kx + C, where k is a constant and C is the constant of integration.

3. Linearity of Integration: Integration is a linear operation. This means that the integral of a sum of functions is equal to the sum of the integrals of the individual functions:∫[f(x) + g(x)] dx = ∫f(x) dx + ∫g(x) dx.

4. Integration of e^x: The integral of e^x with respect to x is itself:∫e^x dx = e^x + C.

5. Integration by Parts: Integration by parts is a technique used to integrate the product of two functions. It is based on the formula:∫u dv = uv - ∫v du, where u and dv are differentiable functions of x.

6. Integration by Substitution: Integration by substitution is used to simplify complex integrals by making a substitution that transforms the integral into a simpler form. The substitution involves replacing variables to simplify the integrand.

7. Trigonometric Integrals: There are several integral rules for trigonometric functions, such as the integrals of sine, cosine, secant, and tangent functions. These rules are used to evaluate integrals involving trigonometric functions.

8. Definite Integrals: Definite integrals involve finding the net accumulation of a quantity over a specific interval. The result is a single numerical value that represents the area under the curve between two points on the x-axis.

9. Integration of Rational Functions: Techniques like partial fraction decomposition are used to integrate rational functions, which are ratios of polynomials.

10. Integration of Exponential and Logarithmic Functions: Integrals involving exponential and logarithmic functions require specific rules and techniques for evaluation.

11. Integration Tables: Complex integrals may require reference to integral tables or computer software to find exact solutions.

These are some of the fundamental integral rules and techniques in calculus. Mastery of these rules and techniques is essential for solving a wide range of mathematical and scientific problems, as integration plays a significant role in various fields of science and engineering.